Verify the Continuity of a Piecewise Function
You should upgrade or use an alternative browser.
- Forums
- Homework Help
- Calculus and Beyond Homework Help
Continuity of piecewise function f(x,y)
- Thread starter jwxie
- Start date
Homework Statement
Determine all points at which the given function is continuous.
For practice, I want to verify the continuity. Moreover, with piecewise function, I have to verify continuity anyway.
Q1
Q2
Homework Equations
The Attempt at a Solution
Let's do the second problem first.
For Q2, we have x =/= y for the first condition, whereas if x = y, we let f(x,y) = 2x.
So to check continuity, we would have to make sure
(1) f(a) is defined,
(2) limit as x goes to a exists, and
(3) f(a) = limit as x goes to a
The piecewise function proved that f(a) is defined for x = y, then we have 2x (for an arbitrary number, possible).
Then I did limit.
limit as y goes to (x = y), we get x^2 - y^2/ x - y, after simplification, we had (x +y) remained. Substituted y = x, we have x+x, so the limit is 2x.
Indeed, f(x=y) = 2x = limit as y goes to x=y
This problem required no squeeze theorem because simplification and substitution worked.
For the first problem, I attempted a few ways.
We know #1 is true, when x^2+y = 1, we have to have f(x,y) = 1.
So I tried to find its limit and see if it would come out to be 1.
So I took limit of the first function. I can't simplify it, so I am down with squeeze theorem and path methods. But I was confused what limit to take. I tried to eliminate one of the parameter, and did L'hospital rule. This allowed me to take the derivative of sin^1/2(...) which would put its derivative with a -1/2 power. Thus I had
-2y (sqrt(1-x^2-y^2)) / -2y cos(1-x^2-y^2) , and cancel -2y.
But I am stuck. If I did x^2 + y^2 = 1 and solve for x (or y), and plug it in, I would always get -x^2 or -y^2 inside the square root, which is not right...
Please tell me whether my approach for Q2 was right, and how to solve for Q1. Thank you.
Answers and Replies
You have the basic idea, but you're being sloppy in your explanation. For example,Homework Statement
Determine all points at which the given function is continuous.
For practice, I want to verify the continuity. Moreover, with piecewise function, I have to verify continuity anyway.
Q1
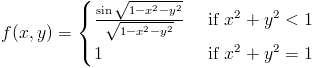
Q2
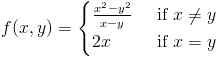
Homework Equations
The Attempt at a Solution
Let's do the second problem first.
For Q2, we have x =/= y for the first condition, whereas if x = y, we let f(x,y) = 2x.
So to check continuity, we would have to make sure
(1) f(a) is defined,
(2) limit as x goes to a exists, and
(3) f(a) = limit as x goes to aThe piecewise function proved that f(a) is defined for x = y, then we have 2x (for an arbitrary number, possible).
Then I did limit.
limit as y goes to (x = y), we get x^2 - y^2/ x - y, after simplification, we had (x +y) remained. Substituted y = x, we have x+x, so the limit is 2x.Indeed, f(x=y) = 2x = limit as y goes to x=y
This problem required no squeeze theorem because simplification and substitution worked.
- f(a) - the function has two variables, not one, so f(a) is meaningless in this problem.
- limit as y goes to (x = y) - I get what you're trying to say, but you're not saying it very well.
[tex]\lim_{(x, y) \to (a, b)} f(x, y)[/tex]
The limit can be written more simply for points on the line y = x.
Typo here. When x^2 + y^2 = 1, f(x, y) is defined to be equal to 1.For the first problem, I attempted a few ways.
We know #1 is true, when x^2+y = 1, we have to have f(x,y) = 1.
??? The function is sin(sqrt(1 - x^2 - y^2)). Where did the sin go?So I tried to find its limit and see if it would come out to be 1.So I took limit of the first function. I can't simplify it, so I am down with squeeze theorem and path methods. But I was confused what limit to take. I tried to eliminate one of the parameter, and did L'hospital rule. This allowed me to take the derivative of sin^1/2(...) which would put its derivative with a -1/2 power. Thus I had
-2y (sqrt(1-x^2-y^2)) / -2y cos(1-x^2-y^2) , and cancel -2y.
Also, you can't just "take the derivative" - this is a function of two variables. You would have to take the partial derivative with respect to either x or y.
Note that your function has two definitions: one for points on the circle x^2 + y^2 + 1, and the other for points inside that circle. That circle can be represented in polar form very simply, and that will help you do what you need to do.
But I am stuck. If I did x^2 + y^2 = 1 and solve for x (or y), and plug it in, I would always get -x^2 or -y^2 inside the square root, which is not right...Please tell me whether my approach for Q2 was right, and how to solve for Q1. Thank you.
Suggested for: Continuity of piecewise function f(x,y)
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Last Post
- Forums
- Homework Help
- Calculus and Beyond Homework Help
perilloandlonimper.blogspot.com
Source: https://www.physicsforums.com/threads/continuity-of-piecewise-function-f-x-y.411744/
Post a Comment for "Verify the Continuity of a Piecewise Function"